Author:
(1) CALLA TSCHANZ.
Table of Links
- Abstract and Intro
- Background on tropical perspective
- The expanded construction
- GIT stability
- Stack perspective
- The canonical moduli stack
- References
5. Stack perspective
5.1 Stacks and stability conditions
Before we describe the expanded degenerations as stacks, we comment on the role of stacks in this problem and on the stability conditions defined in Section 5.3.
Base change. Our aim is to construct degenerations of Hilbert schemes of points as good moduli spaces. In the proofs of Propositions 6.1.2 and 6.1.4, we will use the valuative criterion to prove universal closure and separatedness. We will see that this argument holds only up to base change, which is why it is necessary for us to work with stacks instead of schemes.
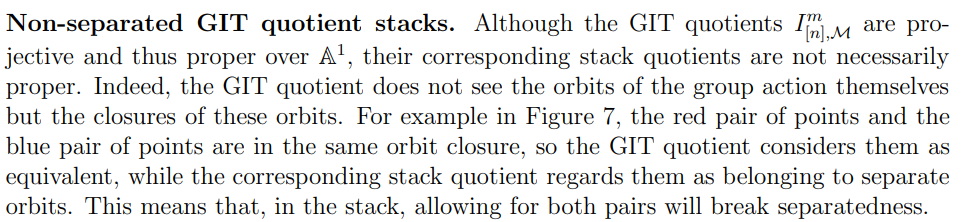
In the following sections, when studying quotient stacks, we will therefore want to consider the sublocus of the GIT stable stable locus containing only length m zero-dimensional subschemes which are smoothly supported in a given fibre of X[n]. Building a compactification in which limits are represented by smoothly supported subschemes will also be useful for future applications as it allows us to break down the problem of a Hilbert scheme of m points on a singular surface into products of Hilbert schemes of fewer than m points on smooth components.

Definition 5.1.1. We say that a fibre in some expanded degeneration X[n] has base codimension k if exactly k basis directions vanish at this fibre. This is independent of the value n.
Making the expanded degenerations large enough. Finally, if we construct a unique GIT quotient in which not all limit subschemes are smoothly supported then the limits given by orbit closures containing only subschemes with singular support will not lie in a fibre of the expected base codimension. This gives an intuition that the degeneration we have chosen is too small. That being said, it can be useful to think about this GIT quotient if what we are trying to do is simply to resolve singularities in a way that preserves some good properties of the space, e.g. in the context of constructing minimal models for type III degenerations of Hilbert schemes of points on K3 surfaces.
5.2 Expanded construction for stacks


5.3 Stability conditions.
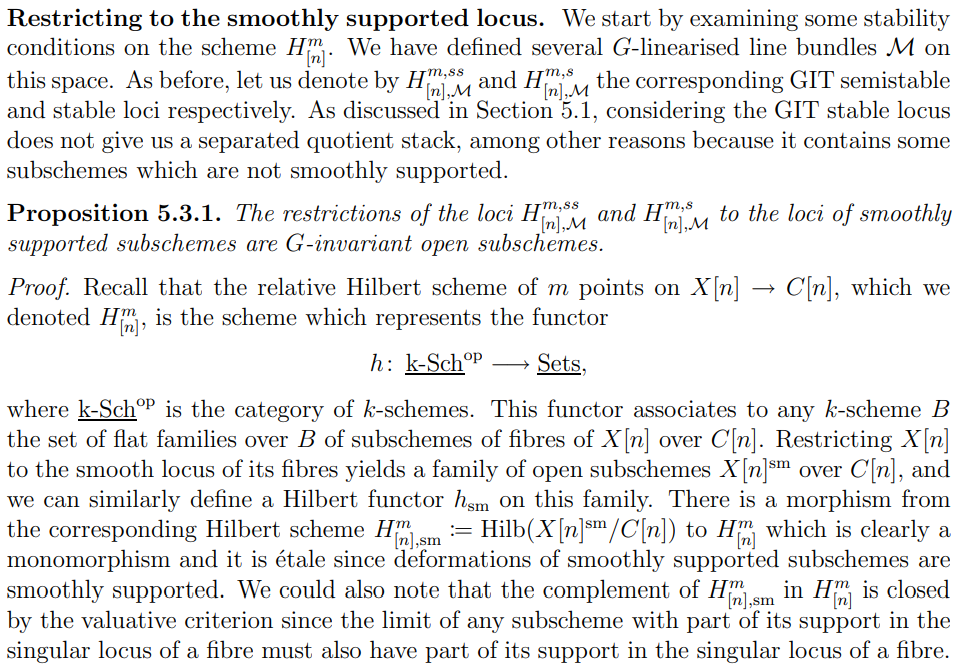
We remark that since the smooth locus of the fibres of X[n] is G-invariant, restricting the functor to this locus preserves the G-invariance. The restrictions of the semistable and stable loci to the loci of smoothly supported subschemes therefore yield G-invariant open subschemes.
We have the following inclusions:
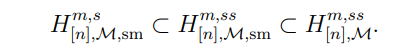
Li-Wu stability. We recall here the notion of stability used in [LW15], in order to compare it with the GIT stability and construct an appropriate stability condition for this case.
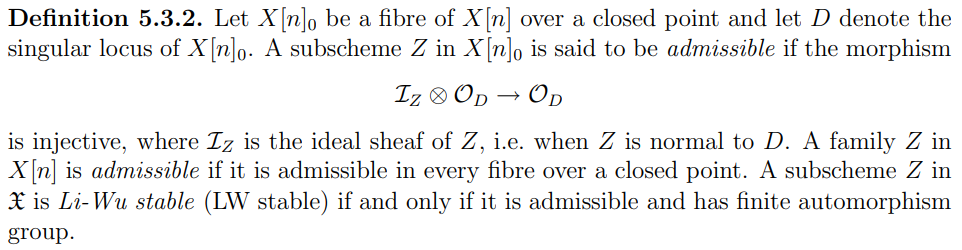
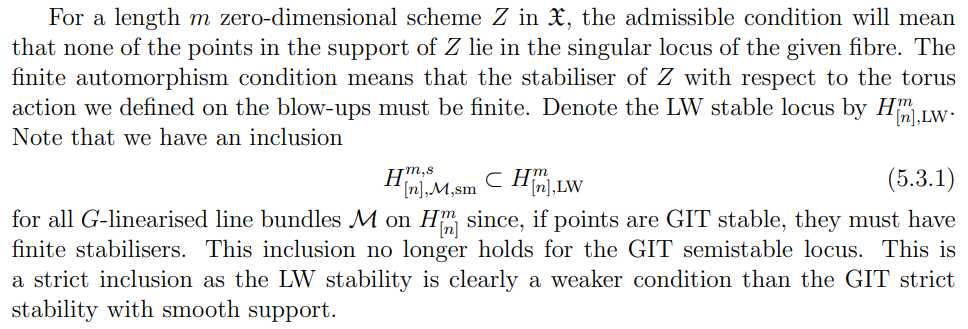
Modified GIT stability. As stated above, we only want to allow length m zerodimensional subschemes to be stable if their support lies in the smooth locus of a fibre. However, restricting the GIT stability condition to this locus makes the space of stable subschemes no longer uiversally closed. Indeed, there is no single GIT condition which can represent all desired length m zero-dimensional subschemes as smoothly supported subschemes. We must therefore define a modified GIT stability condition which patches together several GIT stability conditions in order to obtain the desired stable locus.

This paper is available on arxiv under CC 4.0 license.

